Generalized Simulated-Annealing Optimization for Inversion of First-Arrival Times
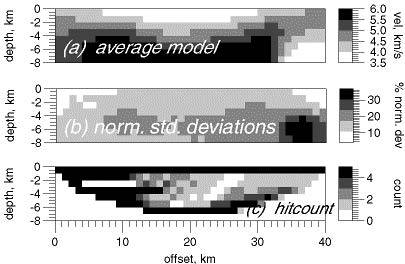
We employ a Monte Carlo-based optimization scheme called generalized simulated annealing to invert first-arrival times for velocities. We use “dense” common depth point (CDP) data having high multiplicity, as opposed to traditional refraction surveys with few shots. A fast finite-difference solution of the eikonal equation computes first arrival travel times through the velocity models. We test the performance of this optimization scheme on synthetic models and compare it with a linearized inversion. Our tests indicate that unlike the linear methods, the convergence of the simulated-annealing algorithm is independent of the initial model. In addition, this scheme produces a suite of “final” models having comparable least-square error. These allow us to choose a velocity model most in agreement with geological or other data. Exploiting this method’s extensive sampling of the model space, we can determine the uncertainties associated with the velocities we obtain.
We use the optimization procedure on first-arrival times picked from two sets of field shot records. One is the data set from COCORP Death Valley Line 9, which traverses the central Basin and Range province in California and Nevada. The other data set is marine reflection data collected by the EDGE consortium across the Hosgri fault, offshore California. In both cases the velocities in the region undergo rapid lateral changes, and our technique images these variations with little a priori information.
